Difference between Active Power, Reactive Power and Apparent Power

Power is among the most important concepts in electrical engineering as it determines how much energy flows from your circuit to whatever device you are attaching. Several kinds of powers clarify their meanings in different ways and with varying connotations. Here, we will discuss what is active and reactive power, the difference between active power, reactive power, and apparent power, and how this affects performance in AC circuits.

What is Active Power?

Active power is the usable or consumed electrical energy in an AC circuit and has units of watt (W) or kilowatt (kW). True power or real power is another name for active power, and it's the kind that actually does useful work in circuit-heating LED lights, light bulbs, whatever.

Rector shows how Armstrong finds active power by multiplying together the root mean square (RMS) values of voltage and current, as well as a parameter called the power factor, some measure for reflecting offset between them. The formula for active power is:

P=V×I×cosϕ

Where P is the active power, V is the RMS voltage, I is the RMS current, and ϕ is the phase angle between the voltage and current.

Active power is the energy actually used in load. Intermediate power is also the power produced by a source of energy, like a generator or battery.

What is Reactive Power?

Reactive power is the alternating current flowing back and forth in an electric circuit. The unit used for reactive power is volt-amperes reactive (VAR) or kilovolt-ampere active (kVAR). R.P., a term also used for imaginary power or wattless power, is the form of energy stored and released by the reactive components within current flowing through an electric circuit--most commonly inductors (inductance) and capacitors (capacitance).

The formula for reactive power is:

Q=V×I×sinϕ

where Q is the reactive power, V is the RMS voltage, I is the RMS current, and ϕ is the phase angle between the voltage and current.

This power is not useful to the circuit, but it must be maintained for the voltage and current levels. Also, reactive power creates inefficiencies and loss of energy by increasing apparent power, which promotes a greater flow of current.

What is Apparent Power?

The apparent power of a sinusoidal AC circuit can be calculated simply as the product of its RMS values for voltage and current. It is measured in volt-amperes (VA) or kilovolt-amps (kVA). Power is called apparent power, complex power, or total gave, and this refers to the net delivered from a source by a line.

The apparent power is found by multiplying the RMS values of voltage and current, regardless of any phase relationship. The formula for apparent power is:

S=V×I

where S is the apparent power, V is the RMS voltage, and I is the RMS current.

Switching power is the total demand of the circuit. It's a latent manuscript that bears the relationship between electricity and temperature: where does the electronic grade switch make sense? It isn't finished yet. What should be next? But apparent power also refers to the kind of power transmitted upon the lines, and it is this that determines how large or high a rating needs an individual component in any given circuit.

Read Also: What Do The Different Colors Mean On Electrical Wires?

Difference between Active Power and Apparent Power?

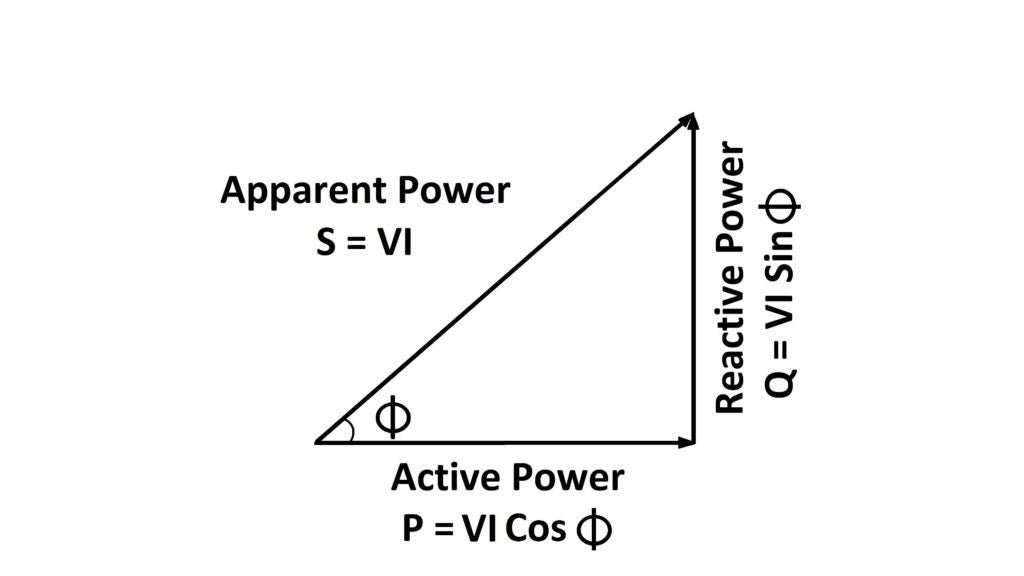
Active power, also known as real power, is the portion of electrical power that is consumed by a circuit and converted into useful work, such as generating heat, light, or mechanical energy. It is measured in watts (W) and represents the actual energy being utilized in a system. In contrast, apparent power encompasses both active power and reactive power, which is the power associated with energy storage and release due to the presence of inductive or capacitive components in the circuit.

Apparent power is measured in volt-amperes (VA) and reflects the total power flowing through the circuit, including both the real power being used and the reactive power required to maintain voltage levels. While active power directly contributes to performing tasks, apparent power accounts for the total power capacity needed in an electrical system.

(Note: θ represents the phase angle between voltage and current in the circuit.)

What is the difference between Active Power and Reactive Power?

The difference between active power, reactive power, and apparent power can be summarized as follows:

• Power equivalence means that the power that is actually absorbed or consumed in the circuit is active, not reactive, electricity. But only some of this active electricity does useful work; other parts return to decrease consumption elsewhere. Reactive power is the power flowing back and forth in this circuit; it's made by itself, stored up, and released. The apparent power supplied to the circuit by the source is called discovered perceived or real power and represents what has been demanded when it comes into contact with resistance.
• Power is measured in watts or kilowatts. It's active power, and it comes with a bill from the utility company. Power factor is usually expressed in terms of kilovolt-amperes or volt-ampere reactive. It's not like the utility company charges for it, though. On the other hand, apparent power is measured in volt-amperes, or kilovolt characters may also punctuate sentences.
• The RMS values of the voltage and current are multiplied by each other and then multiplied again by a power factor to arrive at active power. Its three defining features are RMS values of voltage, current, and the sine of their phase difference. However, apparent power is obtained by multiplying the RMS values of voltage and current. The phase difference between them has no bearing on this calculation.
• The power generated by the power source is active. It's equal to the real part of complex electric energy (complex load). The reactive power is that which gives rise to losses and inefficiencies within the circuit, and it can be expressed as an imaginary part of complex power. The apparent power that flows over the lines is simply the in magnitude, or absolute value of this complex power.

Power and Current Relations

The relation between power and current in an AC circuit can be expressed as follows:

P=I^2×R

where P is the active power, I is the RMS current, and R is the resistance of the circuit.

With this equation, the active power, P, is seen to be proportional both to the square of current and resistance in the circuit. So, if the current goes up, then active power increases by the square of that ambient variable. In a similar way, if the resistance rises passively, power increases by that same amount.

This is also to say that the power and current relation means a larger factor between active power (AC electric energy used) and long lines will move down accordingly, reflecting higher efficiency. The power factor is a way of gauging the efficiency with which reactive energy can be exchanged and how much unwanted reactive energy has to go unabsorbed by us. A high power factor means that our circuit's capability for absorbing this form of fluctuating electric charge comes close to matching its ability (thanks, in part) placed within appropriate limits.

Conclusion

In an AC circuit, there are different kinds of power--active, reactive, and apparent powers. There is some overlap between them in meaning but each has its special implication. The power that works, active power is actually consumed or used in a circuit and really does useful work. Reactive power is the power that flows forward and then backwards in an AC circuit. The reactive components store it up when energy flows to them, releasing it back later as soon as energy demand starts running backwards through the same spot again because of their combination with resonance frequency circuits. The apparent power is passing from the source to the circuit, and this effectively represents what amount of total demand there.

If you are looking for a convenient and reliable way to shop for electrical products and solutions, look no further than the online store of Schneider Electric (SE).